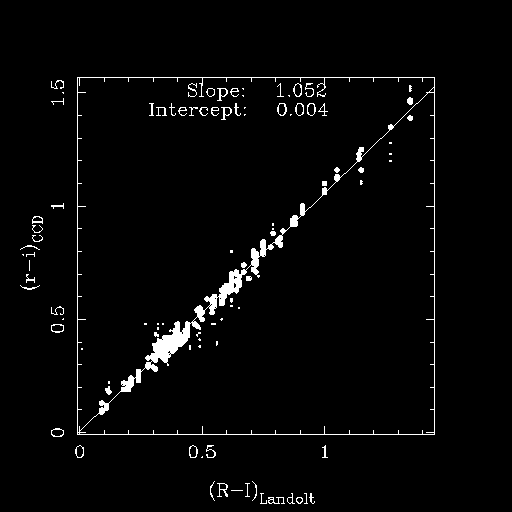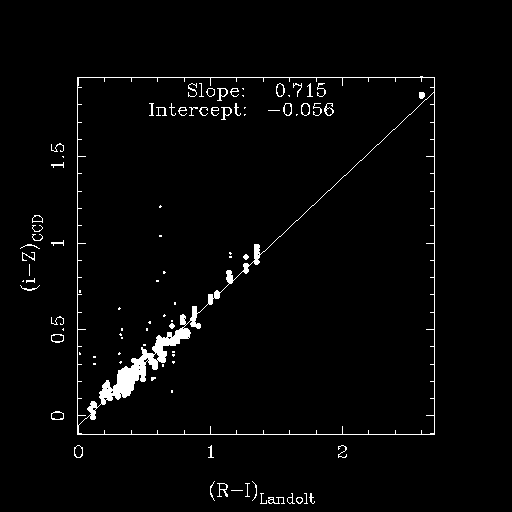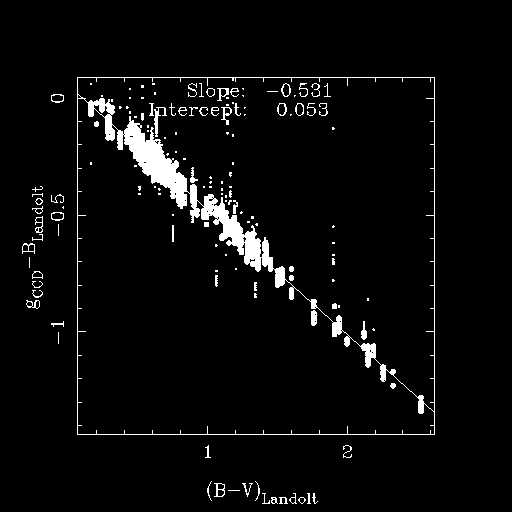Colours and Transformations
Transformations Between Filter Systems
Using WFS observations of Landolt Standard fields (Landolt 1992, AJ
104, 340), we provide relations for tranforming between the natural
WFC system (filter+detector, Vega-like) and this other common
observing systems.
Provisional Colour Terms
| Filter Combination | Landolt Transform | Notes |
|---|
| URGO-ULan | -0.084(U-B)Lan+0.106 |
| BKPNO-BLan | -0.129(B-V)Lan |
| VHarris-VLan | -0.009(V-R)Lan |
| VHarris-VLan | -0.005(V-I)Lan |
| RHarris-RLan | -0.010(V-R)Lan |
| IHarris-ILan | -0.062(R-I)Lan |
| IHarris-ILan | -0.009(V-I)Lan |
| ZRGO-ILan | -0.381(R-I)Lan |
| gCCD-BLan | -0.531(B-V)Lan+0.053 |
| rCCD-RLan | +0.245(V-R)Lan+0.019 | 23/9/03: previous value (-0.245) wrong |
| rCCD-RLan | +0.275(R-I)Lan+0.008 | minor (<1%) change |
| iCCD-ILan | +0.211(R-I)Lan |
| iCCD-ILan | +0.101(V-I)Lan |
Some examples for using these terms in conjuction with the zeropoints are here:
- BCCD = ZPB - 2.5 log10(counts/s) =
BLan - 0.129 (B-V)Lan
- gCCD = ZPg - 2.5 log10(counts/s) =
BLan - 0.521 (B-V)Lan
- ZCCD = ZPZ - 2.5 log10(counts/s) =
ILan - 0.381 (R-I)Lan
Note that the Z-band Landolt zero-point is in the natural WFC filter +
CCD passband and has been approximately normalised to the Vega system
using an extrapolated colour correction for the Landolt photometry.
Transforming between WFS magnitudes and SLOAN AB
The following equations were derived using stellar objects with good
photometry in both SLOAN and WFS in the ELAIS N1 area:
| uAB = u + 0.845 |
| gAB = g - 0.10 + 0.12 * (g - r) |
| rAB = r + 0.10 - 0.02 * (g - r) |
| iAB = i + 0.26 + 0.09 * (r - i) |
| zAB = z + 0.43 + 0.01 * (i - z) |
where uAB,...,zAB are the magnitudes in the
SLOAN system and u,...,z are the magnitudes in the WFS system (Vega),
i.e.,
u = ZPu - 2.5*log10(counts/s)
Transforming Colours
Using standards measured over five runs with good conditions in Jul
1999, Sep1999, Oct1999, Jun2000 and Sep2000 we find the following
colour-equations to transform between the Landolt (Johnson-Cousin's)
and WFS natural filter+ccd (Vega) systems.
Please exercise caution when using these relations, they
have not been thoroughly tested, and do not yet include error
analysis. For example the offsets in the relations should be equal to
zero (i.e the case for an A0 star with colour defined to be zero
between all passbands). I'm not yet sure whether these values are
within error.
(g-r)CCD=0.908(B-V)Lan+0.048
(r-i)CCD=1.052(R-I)Lan+0.004
(i-Z)CCD=0.715(R-I)Lan-0.056
(URGO-BKPNO)CCD=1.022(U-B)Lan+0.156
(BKPNO-VHarris)CCD=0.904(B-V)Lan+0.004
I've saved the U-g colour till last as it's the most complicated
given there is a degeneracy in U-B (and U-g) around the balmer
jump. The following prescriptions seem to work reasonably well and are
in reasonable agreement with data where there are sufficient blue
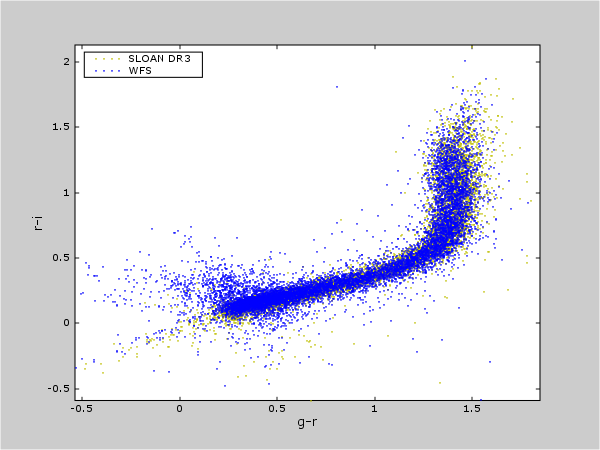
stars (see colour-colour plot at bottom of page).
| (U-g)CCD=1.271(U-B)Lan+0.37 |
(for B-V>0.5) |
| (U-g)CCD=1.271(U-B)Lan |
(for 0.5>=B-V>0) |
| (U-g)CCD=(U-B)Lan |
(for 0.0>B-V) |
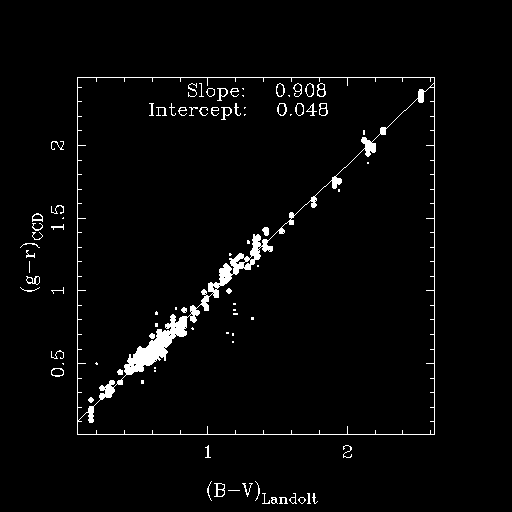
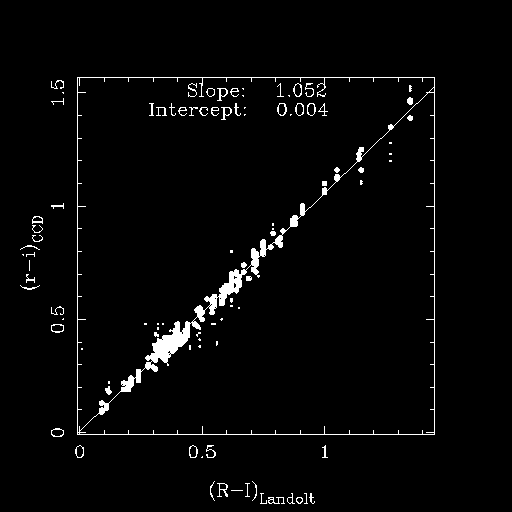
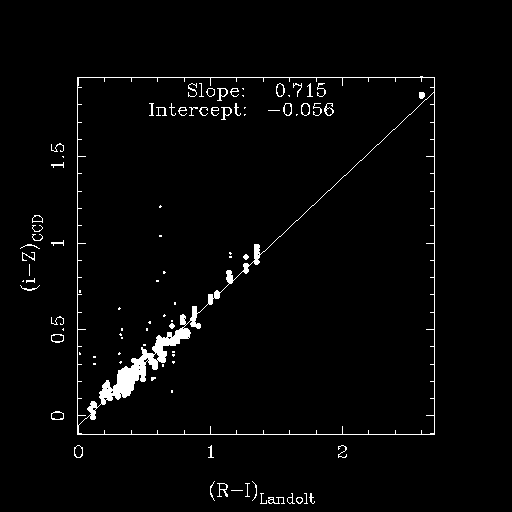

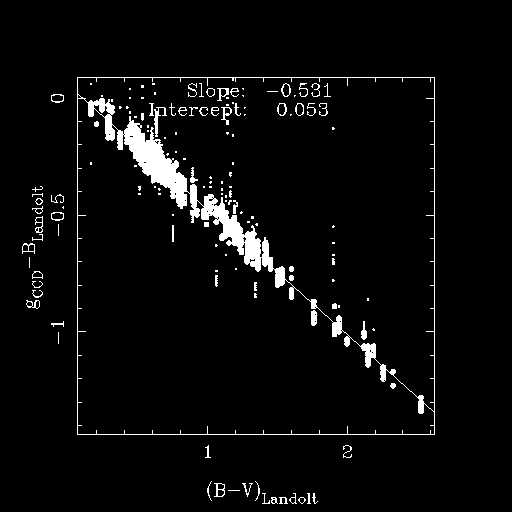
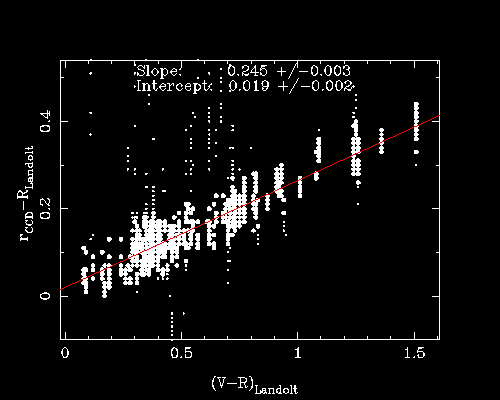
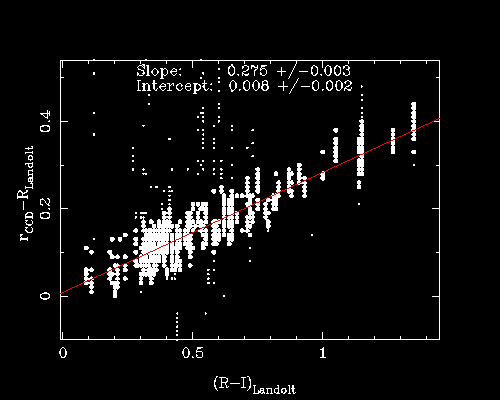
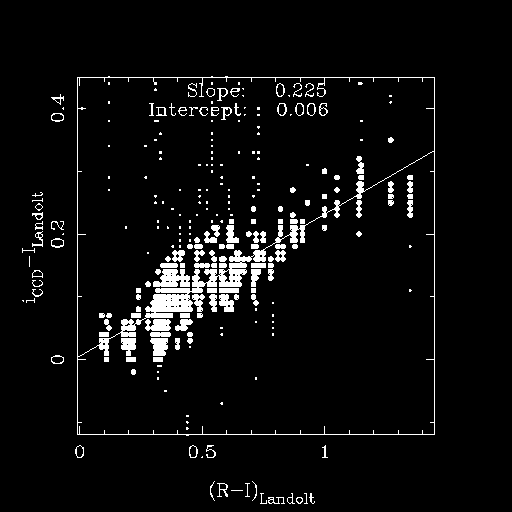
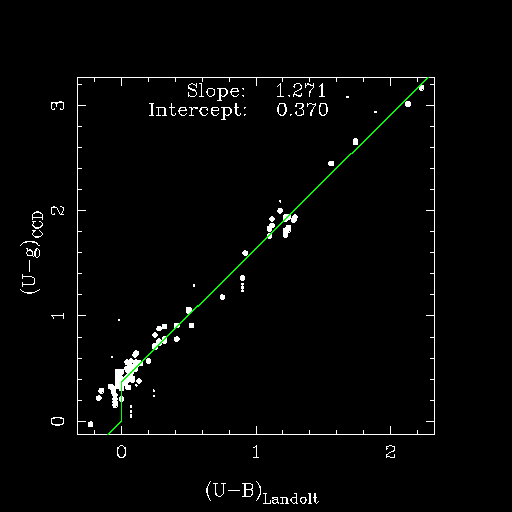
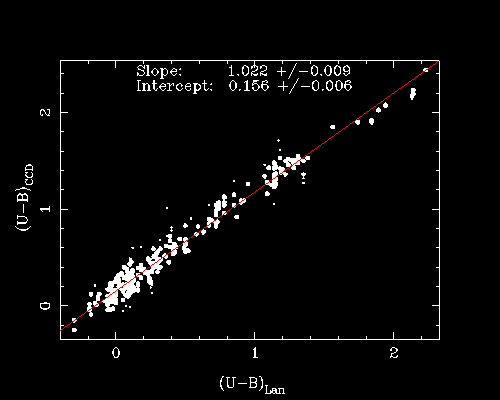
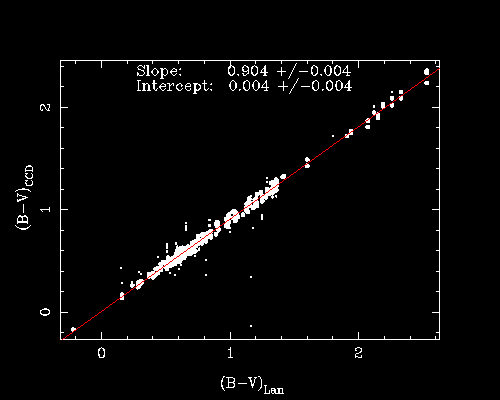
The following data are from WFC imaging of the Ursa Minor dwarf
galaxy which shows a nice horizontal branch. This isochrone is from
the Hyades UBV data and converted to Ugr using the relations above.