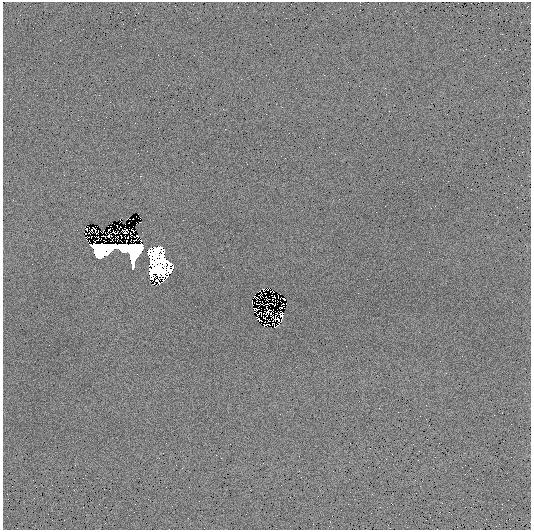
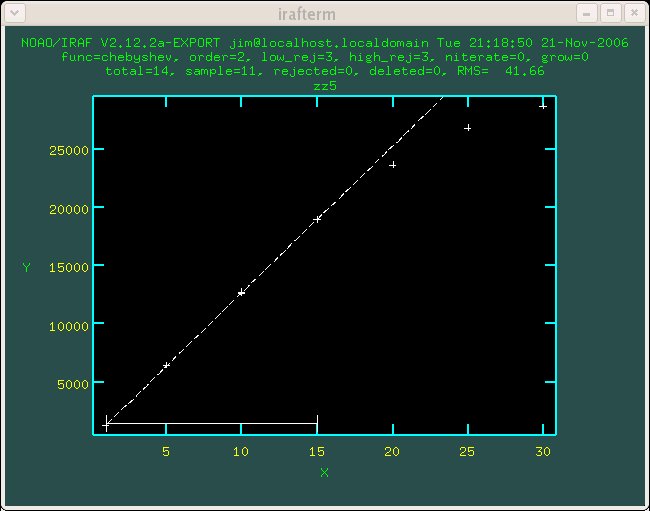
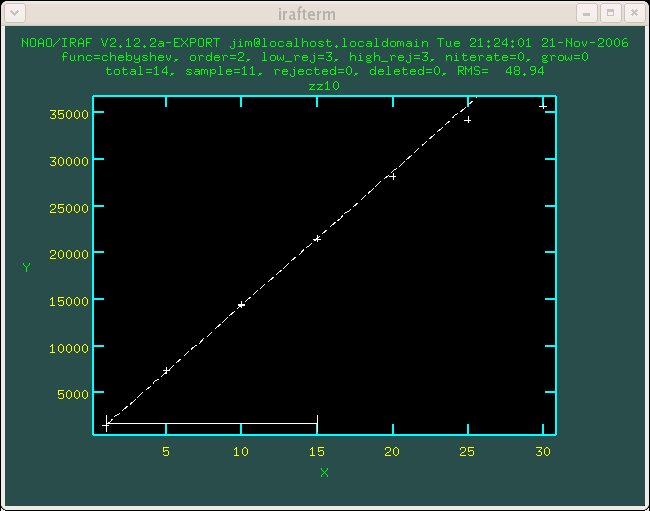
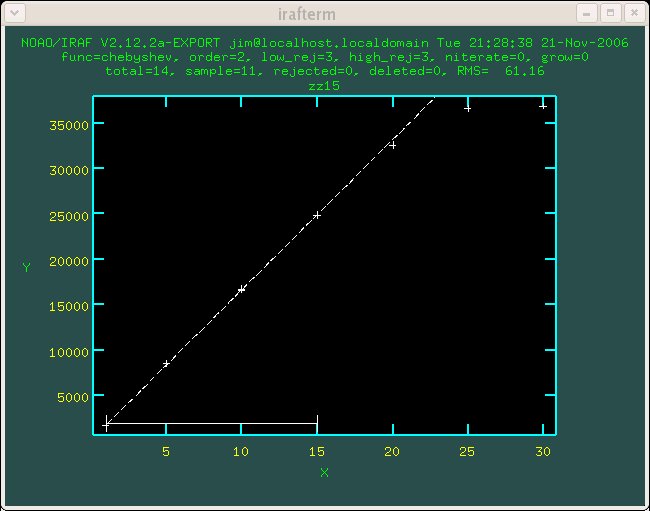
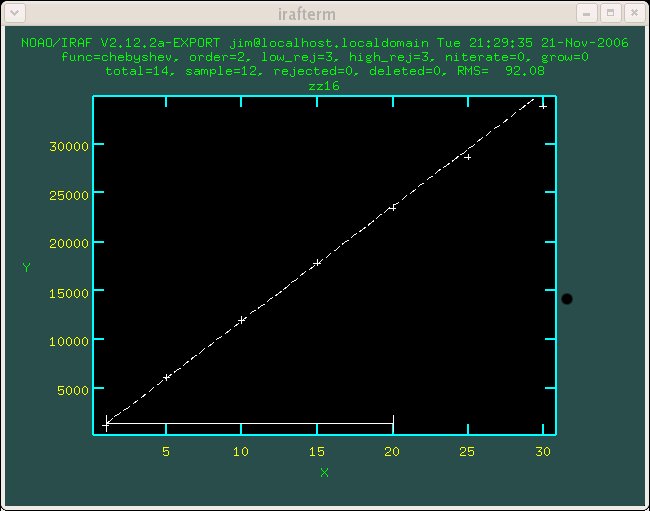
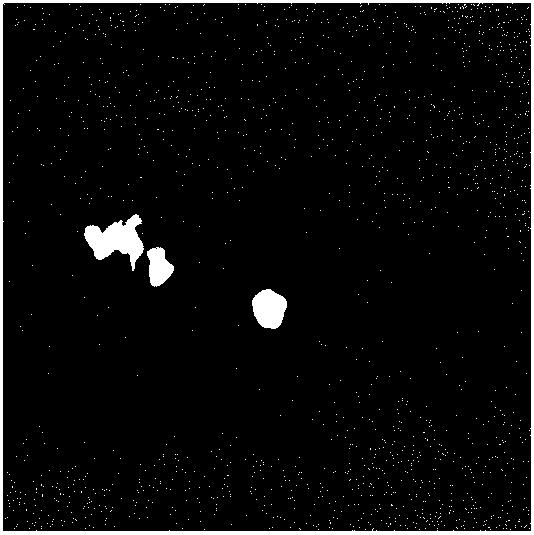
Figure 1.1 Detector 1

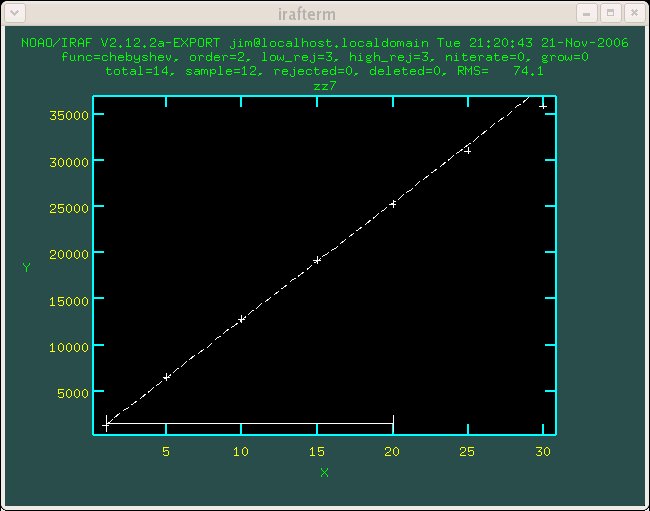
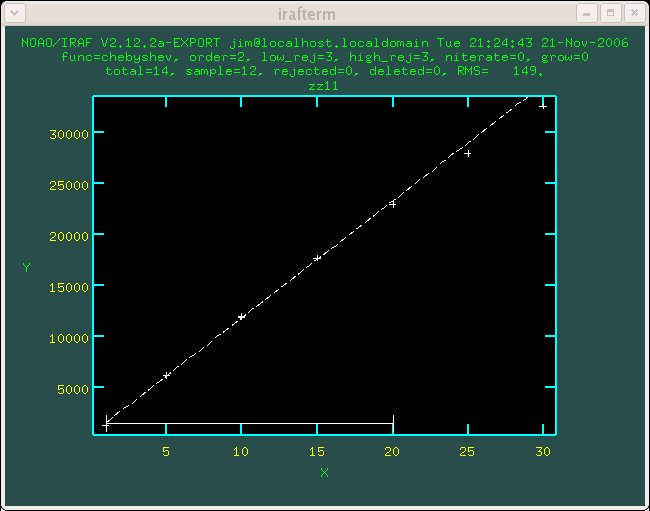
Figure 1.2 Detector 2

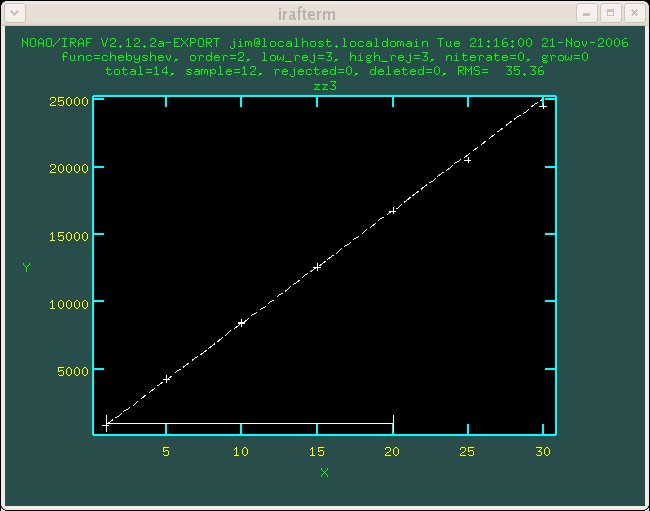
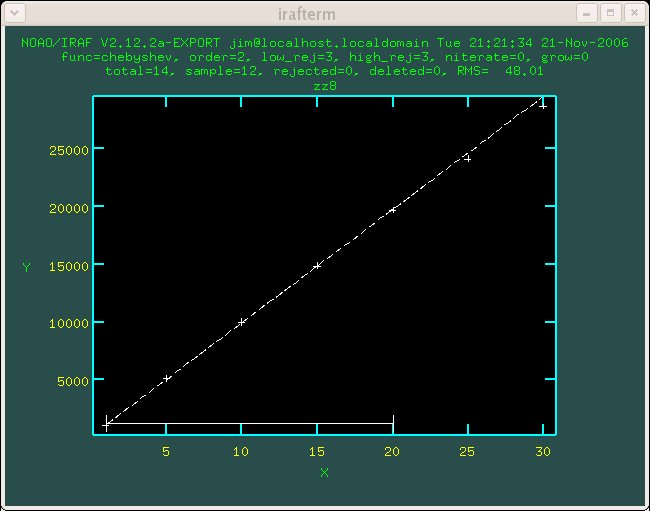
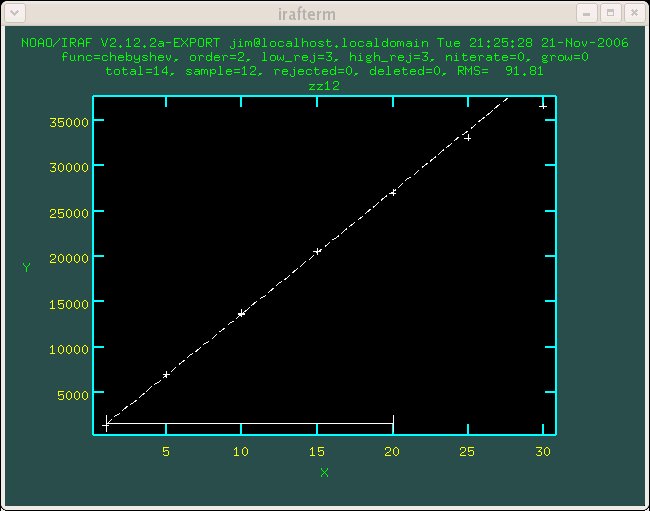
Figure 1.3 Detector 3
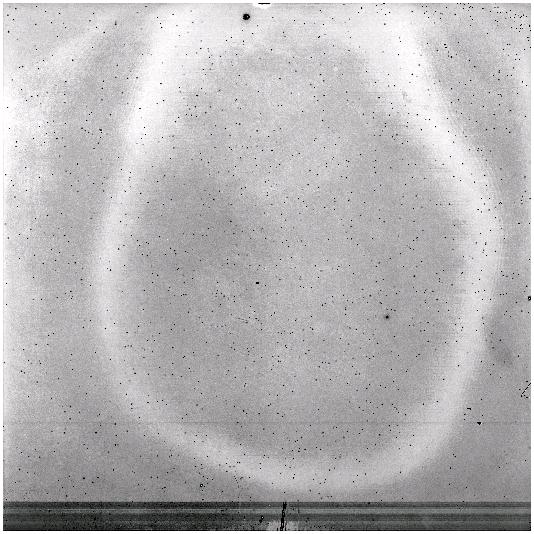
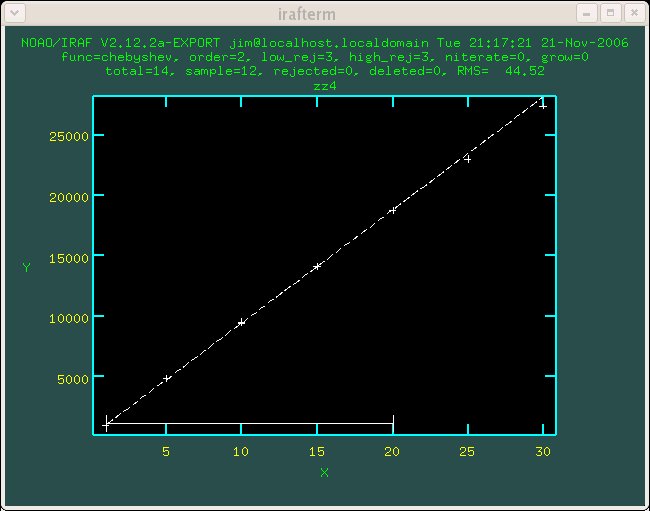
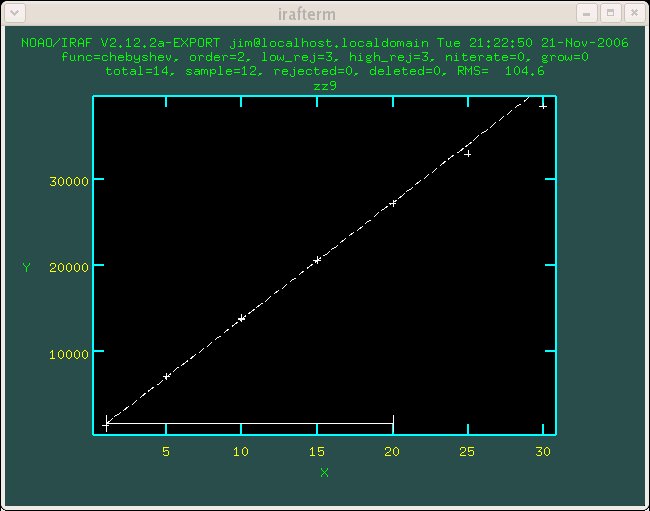
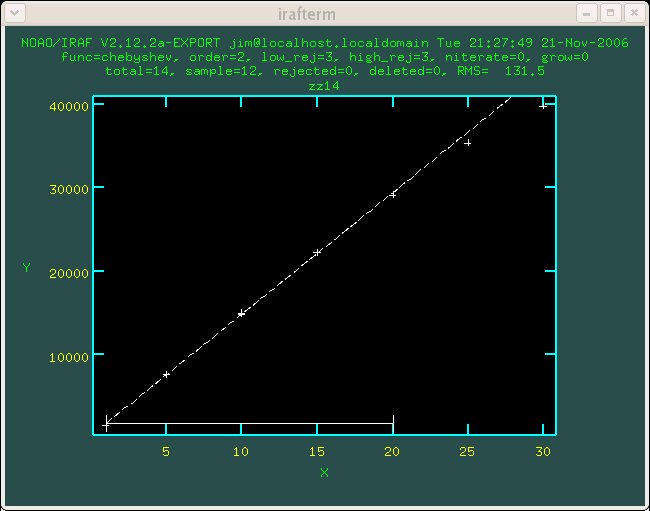
Figure 1.4 Detector 4
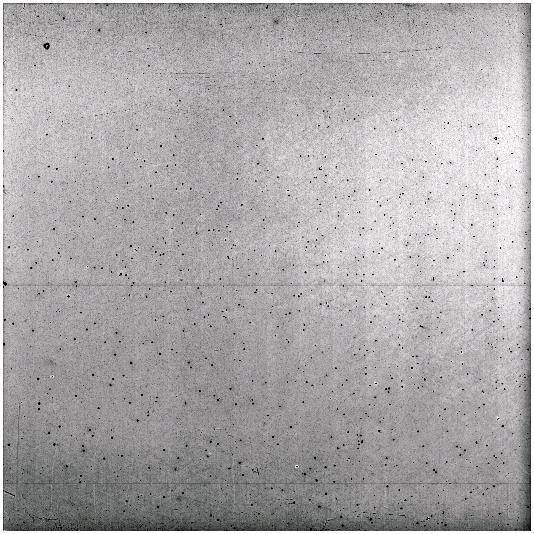
Figure 1.5 Detector 5

Figure 1.6 Detector 6

Figure 1.7 Detector 7

Figure 1.8 Detector 8

Figure 1.9 Detector 9

Figure 1.10 Detector 10

Figure 1.11 Detector 11

Figure 1.12 Detector 12
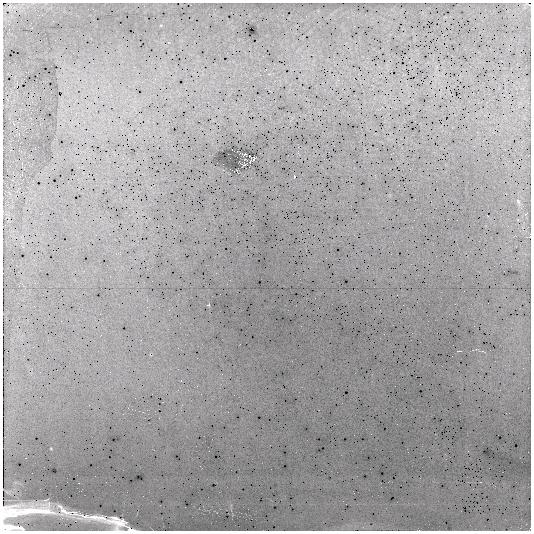
Figure 1.13 Detector 13
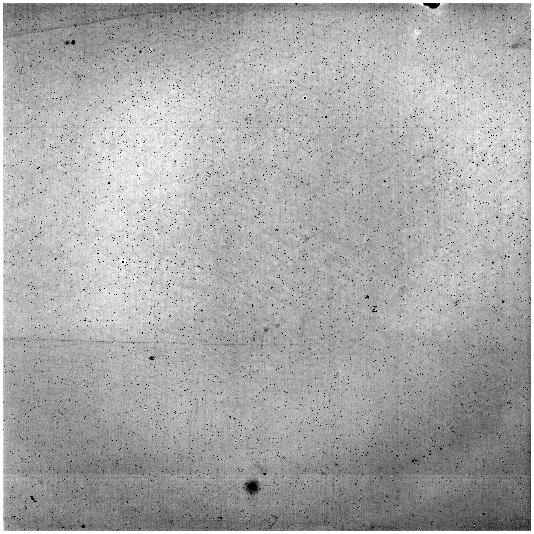
Figure 1.14 Detector 14
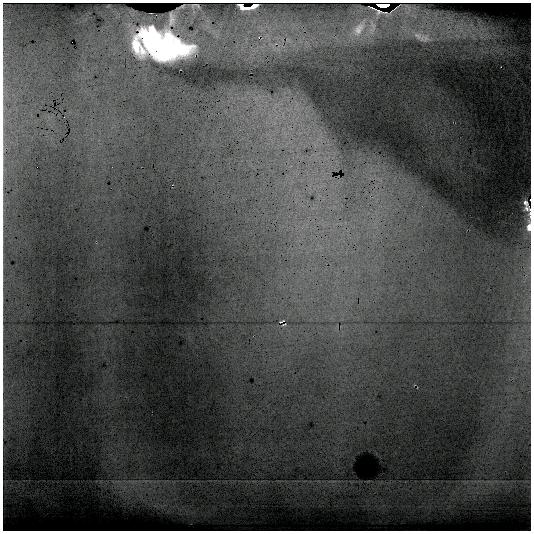
Figure 1.15 Detector 15

Figure 1.16 Detector 16