UFTI Service Observation Reductions

(Document number VDF-TRE-IOA-00008-0001)

Jim Lewis

Introduction

Over the past several years we have been trying to make sense of the best way to reduce data from WFCAM. As NIR data differs from optical data in various ways, it is not always possible to apply tried and tested methods from the optical to the infrared. All cameras are different, so the only way that we'll be able to determine the optimal way to deal with WFCAM data is to wait until some WFCAM data becomes available. While we can begin this process for WFCAM with laboratory test data, final tuning will require on-sky characterisation. Meanwhile we can use data from other infrared cameras to test various options. Data from UKIRT's imager UFTI is the subject of this report.

Data reduction of UFTI data has been going on for some time and there are standard recipes in place that cover the various observing modes and object types. Over the past few years, in designing and implementing a data processing pipeline for WFCAM, we have noticed several aspects of UFTI data reduction that we have found a little unsatisfactory (we have spoken about this at length in the past). As a reminder, some of the worrying issues are:

Dark correction

Currently this is done by taking a single dark frame before a given set of exposures. This is subtracted off of each target frame as the first major reduction step. Ideally the mean 2d dark current signature should be derived from many frames that have been averaged. This allows cosmic rays and other defects to be rejected and hence not propogated through the target data and also the reduces the additional dark frame rms pixel noise. It is also the case that a dark frame on UFTI is not so much a measure of dark current, but also an estimate of the reset anomaly. All IR detectors seem to display this to some degree and it has been seen in most of them to vary with exposure time (this is very obvious in ISAAC data for example).

Flat fielding

Flat fielding can be done in a number of ways, none of which are currently 100% satisfactory. The most popular options are:

Twilight sky flats: These are good in that the flux levels are high enough to ensure good statistics and illumination levels should be pretty uniform. The high flux levels are also useful in making sure that effects due to fringing and dust do not dominate the background. The colour match to the night sky is not ideal, but is fairly close. Time to observe these is limited and it may not be possible to observe flats in all the filter passbands on a single night. (This is not an issue if the flats are stable over longer time periods.)
Dome Flats: The flux levels can be adjusted to whatever you wish. There are problems with uniform illumination and the background sky colour match is bad. They can be observed during the day, so acquiring them is simple and, if nothing else, they can be used for deriving and monitoring bad pixels.
Night sky flats: The main benefit of these is that they are derived from night sky observations and hence the colour match to the target frames is very good. Illumination is uniform too. The count rates are relatively low however and hence the sky signature is dominated by fringing and thermal dust emission. With UFTI the fringing can be as much as 6% (peak to trough), which could result in a similar sized systematic error in photometry.

From these considerations, it is reasonably clear that twilight sky flats give the best chance for an accurate representation of the 2d gain profile of the detector.

Background variations

With the term 'background' here we are coalescing several additive effect like thermal dust emission, 2d sky background and fringing (and residual reset anomaly if present). All of these can vary and not necessarily sychronously.

In what follows I describe some of the reasons that UFTI data are reduced as they are and how this contrasts with how we think WFCAM data should be processed.

Twilight Flat Problems

Apart from problems of actually obtaining twilight flat exposures (owing to time pressures at the beginning and end of the night), the main reason that these are not used for UFTI seems to be a form of image persistence which appears on the UFTI detector after exposure to a high flux background. Below is an example of what happens to a dark frame after several exposures of a bright twilight.

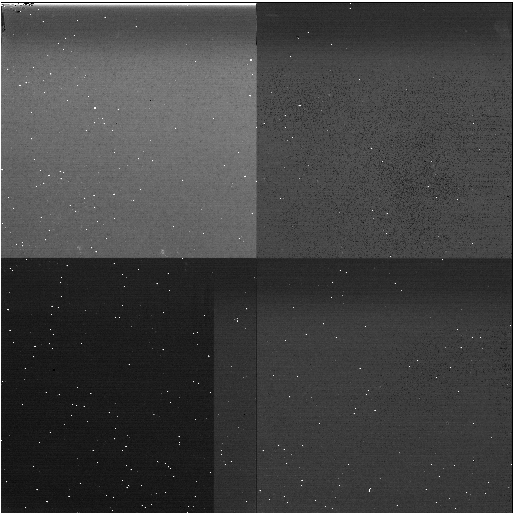
f20030923_00312

The lower lefthand quadrant shows the most worrying trend. These features do decay with time though and return to near normal within 10 to 20 minutes. The actual twilight flat field exposures begin to show unusual features once the count rate gets above about 1200 counts per second. Below is an example of a flat field exposure with a count rate of about 1600 counts per second.

f20030923_00311

(NB: The root cause of these effects is not completely clear, but since they are present, they have to be worked around.)

The effects you see in these two exposures have lead us to the belief in the past that doing twilight flats with UFTI is not feasible. On the one hand, if a bright sky is going to affect the detector with some form of persistence that lasts into the night, then at best we would waste up to 30 minutes or so just waiting for the chip to stabilise and this is something that most observers would not be willing to put up with. There is also the issue of what the actual twilight exposures would look like. If taken during sunset, the brightest sky exposures will resemble the above and subsequent exposures (although taken with a low enough count rate) will be affected by the above mentioned persistence, rendering them useless.

The key to resolving this issue is to realise that using twilight exposures taken during sunrise will only show the above features at the end of the run of exposures. Hence any image with a low enough count rate should be useable as these features only show up once the flux reaches a certain threshold. Below is an example of a sunrise twilight flat taken with a count rate of about 850 counts per second.

f20030923_00295

This shows the well illuminated good signal-to-noise flat field that one would like to have. This is a exposure in H, which is a band that has a high amount of fringing. It is worth noting that there is no sign of fringing here, which shows that the background level is high enough to dominate the scene. Although thermal emission from dust in H is not expected you can actually see where the dust has settled on the cryostat window as some of the dark spots.

Given a series of flat exposures like this, it would be possible to use twilight flats with UFTI. This then gives us hope of flat fielding data from WFCAM in a similar way (ie. derived external to the observing blocks). The only real difference is the projected sky area on the pixels for WFCAM, which gives a smaller window of opportunity before saturation effects kick in.

Dark Correction Problems

As mentioned before, the standard procedure with UFTI is to take a single dark exposure before observing the current exposure sequence. This dark exposure is taken with the same exposure time as the target images and hence both the dark current and reset anomaly for the target frames should be well modelled by it (other caveats notwithstanding). Using a single dark frame to do this correction means that transient defects in the dark frame propogate into the target frames. Whereas a mean dark frame that has been formed from many individual exposures (with appropriate exposure times) can have such things filtered out and the rms noise reduced. If a set of exposures must have its own mean dark frame, as seems to be the case, then to do a series of dark exposures for each target exposure series would cause a serious drop in observing efficiency.

It is often the case in the infrared that it is highly desirable to subtract a 2d sky estimate. This helps to remove fringing and thermal background variations and seems pretty much essential for UFTI. Given that and the existence of an independent mean flat field (i.e. a flat not formed from a combination of the current target frames), a better option might be not to do a separate dark correction step on the target frames at all. Since the sky background, the reset anomaly and the dark current are all additive terms they can all be subtracted together after the target frames have been flat fielded. Doing a combination of the post flat-fielded target frames (with suitable rejection so that stars and transients are removed) will lead to a frame that is a combination of the mean sky, reset anomaly and dark current. This one frame can be subtracted from the from each of the target frames in turn to remove all three effects.

Extra Background Variation Removal

In the previous section I mentioned that using a combination of all post flat-fielded target exposures would model out not only the dark current and the reset anomaly, but also the sky background. The latter is not strictly true when it comes to removing contributions from things like thermal dust emission and fringing.

Thermal dust emission in UFTI comes from radiation emitted by dust on the cryostat window and is only visible in the K band. The flux depends on temperature and thus if the latter is stable, so is the former. This means that although the mean sky background level may vary, so long as the dust temperature is reasonably stable, then the amount of thermal emission won't vary much.

Fringing in UFTI appears to be visible in all three of the main broad band filters, but especially in J and H. Below are sky exposures in J and H (the latter has been flat fielded)

f20020428_00103 to 00111

f20030923_00006 to 00050

Each of these exposures clearly shows two sets of fringes. The exact origin of these is not at issue here. We need to accept that (1) they exist and (2) are not part of the flat field (which the flat field exposures earlier on in this report clearly show).

Below is image of the sky in K. The fringes that look like concentric rings in the previous two wavebands are still present here, although the centre seems to have shifted somewhat. All of the fuzzy dots you see are caused by thermal emission from dust.

f20031008_00153 to 00201

The problem with fringes is that they can vary over the night and within one exposure series in the same way that the mean background does, but not necessarily sychronously with it. If the fringing varies over timescales shorter than that of a single exposure sequence (that is the timescale of the exposures used to form the mean sky frame) then it will be necessary to put in an extra defringing step to correct for this. In what follows, I have just done a simple subtraction of a single mean sky frame for each exposure series. The fringing is removed to a very high degree, but in some frames a residual is visible. I'll assess the level of this residual later on.

Observations and Adopted Reduction Procedure

Two nights of UFTI observations done on 20030923 and 20031008 are used in these examples. They were taken in service time to be used by the groups working on WFCAM data processing to help decide the best way to reduce WFCAM data. Only H and K observations were done during these nights, and as such I'm reducing the scope of this analysis to those two wavebands only. The observations consist mainly of long (35-40) minutes exposure sets, each exposure being between 10 and 40 seconds long.

The reduction procedure employed and outlined below represents what I feel will get the best result from these data sets. This is not to say that this is the definitive way to reduce data from WFCAM.

Form a mean flat field. Choose sunrise twilight flat field exposures where the count rate is high enough to dominate the fringing and thermal emission, and low enough not to show the persistence problems demonstrated above. In the case of both of these nights, a single dark frame was taken before the twilight exposure series and this was used to dark correct the twilight flats. The ideal would be to take a series of darks just before sunrise to fulfill this purpose (a series of darks taken after sunrise would obviously not be useful). Combine the dark-corrected twilight flat field frames into a mean frame using a suitable rejection algorithm to remove transient remnants. Normalise this frame to a mean of 1.0. Create an initial confidence map from the flats.
Divide each target frame in an exposure series by the flat field. Then combine all the target frames in the series to form a mean sky image (again with suitable rejection to remove transients and astronomical objects). Normalise this average sky frame to an mean value of zero.
Subtract the mean sky frame from each of the flat fielded images in the exposure series. Having normalised the mean sky frame to zero ensures that the DC sky level is retained in each object image.
Assign a rough WCS to each target frame based in header information. Use this information and the positions of objects on the frames to work out the cartesian offsets between images.
Combine the object frames using the derived offsets into a single stacked image. Do weighting using the confidence map.
Generate a catalogue of objects on the stacked image. Use this catalogue to fit a WCS to the stacked image and hence assign equatorial coordinates to each object. (Astrometric standards come from the 2MASS point source catalogue.) Objects in the catalogue are also classified as stellar/non-stellar/noise.
Work out a photometric zero point.

Results

Below are some of the tiles that have resulted from these service nights.

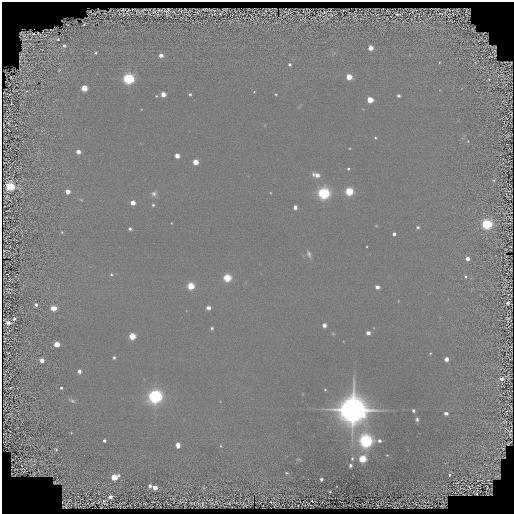
Tile 5 from 20030923 (H)

Tile 60 from 20030923 (H)

Tile 98 from 20031008 (K)

Tile 144 from 20031008 (K)

Discussion of Results

Without an accurate transformation of 2MASS magnitudes onto the UFTI system, it's impossible to work out a real photometric zero point from these observations. Using the naive assumption that they are the same, then the zero points from stars on both nights agree with 2MASS to a rms of 0.1 magnitudes. This is well within the limits constrained by the internal consistency of the 2MASS magnitudes, especially at the faint end. The astrometric accuracy works out at about between 90 and 100 milli-arcseconds. Comparing a set of frames reduced this way with the same frames reduced with night sky flats also shows that using twilight flats reduces the mean sky noise in the individual frames by about 4-5%.

There appears to be a type of pickup noise which is just visible in tiles 60 and 144. The pickup appears on some frames at about the 0.5 to 1% level, but not on others. When it appears the pattern location appears to vary, so this is not an artifact of the reduction process. If this sort of thing appears on WFCAM frames, then perhaps a background subtraction algorithm where the mean background is formed from a sliding median of surrounding frames would be the best way to remove it.

There is one other issue of further concern related to whether the processing accurately removes the fringing that has been seen in all the frames. The fringe removal was only done during the sky background subtraction phase and if the fringe pattern varies a lot over the course of the series of exposures, then there may will be positive or negative fringe residuals on the individual frames. Looking at the images that combined to make tile 5 from the night 20030923, we look at the difference in the median in two regions to work out the amount of fringing on each frame before and after sky subtraction. On this particular tile, the average fringing was 3.12% of the sky background. The dispersion about this mean was 0.13%. After sky subtraction the average difference was 0.01% with a dispersion of 0.14%. Below is one of the images in this series with the largest amount of fringing. After it, is the same image after sky subtraction. Certainly to the eye there is virtually no residual fringing to be seen.

f20030923_00014 flat fielded

f20030923_00014 flat fielded and sky subtracted

Last modified: Mon Feb 16 16:52:01 2004